Mechanics - The ultimate problems for CBSE/NCERT/ IIT -JEE (Main+Advanced) and NEET
Mechanics
Part (A) Core
- Kinematics
A. Vectors
B. Straight Line Motion
C. Motion in Plane (Projectile & Circular Motion)
- Newton's Laws of Motion (NLM)
- Friction
- Work Energy Power
- Centre of Mass (C.O.M.) & Collisions
- Rotational Mechanics (Dynamics of Rotational Motion)
(Part -B) Applied
-Gravitation
- Mechanical properties of solids
- Fluid Mechanics
- S.H.M. (Simple Harmonic Motion)/Oscillation
- Waves (Wave Mechanics)

Q1.A solid disc is rolling without slipping on a horizontal ground as shown in figure. Its total kinetic energy is 150 J . Its translational and rotational kinetic energies respectively are
50 J , 100 J
100 J , 50J
75 J, 75J
125J , 25J

Q2.For given system of two blocks, find the maximum value of force F which can be applied on the system as shown in figure so that both blocks move together. [Given coefficient of static friction between both blocks = 0.3]

Q3.What is the maximum compression in the spring, if the lower block is shifted to rightward with acceleration a. (Given that all surfaces are smooth as shown).
\frac{ma}k
\frac{2ma}k
\frac{mak}2
\frac{ma}{2k}

Q4.The density of a rod AB increases linearly from A to B. Its mid-point is O and centre of mass is at C. Four axes pass through A, B, O and C , all perpendicular to the length of rod. The moments of inertia of the rod about these axes are I_{A}, I_{B}, I_{O} and I_{C} respectively. Then
I_{A}> I_{B}
I_{C} < I_{B}
I_{O}> I_{C}
All of these
Q5. Moment of inertia of a uniform rod of length L and mass M, about an axis passing through L/3 from one end and perpendicular to its length is
\frac2{27}M\;L^2
\frac1{6}M\;L^2
\frac1{12}M\;L^2
\frac19M\;L^2
Q6.A ball of mass m moving at a speed v makes a head on collision with an identical ball at rest. If the kinetic energy of the balls after collision is \frac34th of the original. Then the coefficient of restitution is
e=\frac1{\sqrt2}
e=\frac12
e=\frac{\sqrt2}3
e=\frac13
Q1.F-x equation of a body of mass 4 kg in S.H.M. is F+\;16x\;=0.Here, F is newton and x in meter. The time period of oscillations will be
6.28\; sec
3.14\; sec
1.57\; sec
1 \; sec
Q2. A spaceship is launched into a circular orbit close to the earth's surface. What additional velocity has now to be imparted to the spaceship in the orbit to overcome the gravitational pull. Radius of earth =6400 km , g= 9.8 ms^{-2}
3.2 km/s
11.2 km/s
1.5 km/s
Q3.A geostationary satellite is orbiting the earth at a height of 6R above the surface of earth where R is the radius of earth. The time period of another satellite at a distance of 3.5 R from the centre of earth is
16.96 hours
8.48 hours
9.25 hours
15.76 hours
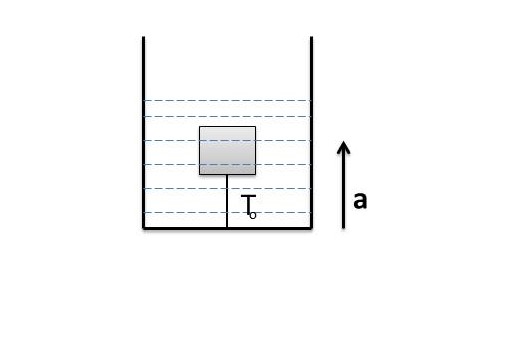
Q1.The tension in a string holding a solid block below the surface of a liquid (of density greater than that of solid) as shown in the figure is T_o when the system is at rest. What will be the tension in the string if the system has upward acceleration a.
T_o\frac ag
T_o\left(1-\frac ag\right)
T_o\left(1+\frac ag\right)
T_o\left(\frac ag-1\right)
Q2. A small body of density ρ is dropped from rest at a height h into a lake of density σ , where σ > ρ . What would be of the acceleration of body till it moves inside the lake? (Neglect all dissipative effects)
g\left(\frac\sigma\rho-1\right)\;downwards
g\left(\frac\sigma\rho-1\right)\;upwards
g\left(\frac\sigma\rho\right)\;downwards
g\left(\frac\sigma\rho\right)\;upwards
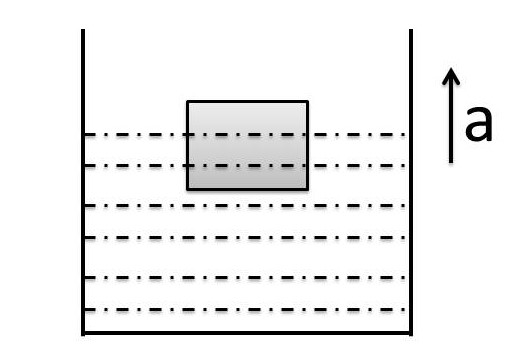
Q3.A partially immersed solid block of \rho_s is floating in a liquid of density \rho_L as shown in figure. If beaker container moves up with positive acceleration 'a' , what is correct statement about the block?
It sinks more inside the liquid
It sinks lesser inside the liquid
It neither sinks more nor lesser inside the liquid
Can not be predicted as data is insufficient
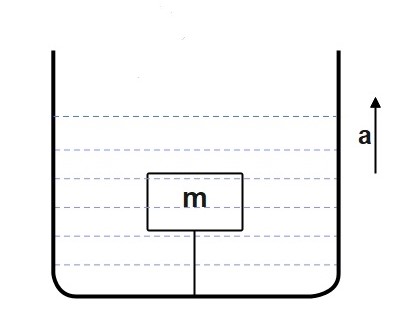
Q4.A block of mass 1 kg and density 0.8 g/cm^3 is held stationary with the help of a string as shown in figure. The block is completely immersed into the water filled in tank.If the tank is accelerating vertically upwards with an acceleration a = 2m/s^2. Find the tension in the string.
Q5.A wooden ball of density 900 kg / m^{3} is immersed in a liquid of density 1200 kg / m^{3} to a depth of 126 cm and the released. The height h above the surface of which the ball rises will be

Q6.A capillary tube of radius r is immersed in water and water rises in it to a height h. The mass of water in the capillary tube is 4g. Another capillary tube of radius 2r is immersed in water. The mass of water that will rise in this tube is:

